Class 7 Maths Ganita Prakash Chapter 2 Arithmetic Expressions NCERT Solutions
Class 7 Maths Ganita Prakash Chapter 2 Arithmetic Expressions NCERT Solutions cover exercises and questions of Chapter 2 from the new textbook Ganita Prakash for 2025-26. These solutions are intended to help the students find practical solutions to the Chapter Arithmetic Expressions. These solutions are designed to solve problems straightforwardly, and diagrams, wherever required, are used to simplify the problems further.
Class 7 Maths Ganita Prakash Chapter 2 Arithmetic Expressions NCERT Solutions Questions and Answers – PDF Download
Page 24
Q. Example 1: Mallika spends ₹25 every day for lunch at school. Write the expression for the total amount she spends on lunch in a week from Monday to Friday.
Solution:
Money spent every day = ₹25
Expression for the total amount spent from Monday to Friday = 5 × 25.
Q. Choose your favourite number and write as many expressions as you can having that value.
Solution:
Let the number be 7.
Some expression with 7:
12 – 5 = 7
20 – 13 = 7
42 × 6 = 7
7 × 1 = 7
3 + (9 – 5) = 7
(26 – 13) – (10 – 4) = 7 etc.
Page 25
Figure it out
1. Fill in the blanks to make the expressions equal on both sides of the = sign:
(a) 13 + 4 = ____ + 6
(b) 22 + ____ = 6 × 5
(c) 8 × ____ = 64 ÷ 2
(d) 34 – ____ = 25
Solution:
(a) 13 + 4 = ____ + 6
17 = ____ + 6
17 – 6 = ____
or ____ = 17 – 6
____ = 11
(b) 22 + ____ = 6 × 5
22 + ____ = 30
____ = 30 – 22
____ = 8
(c) 8 × ____ = 64 ÷ 2
8 × ____ = 32
____ = 32/8
____ = 4
(d) 34 – ____ = 25
34 – ____ = 25
34 – 25 = ____
9 = ____
or ____ = 9
2. Arrange the following expressions in ascending (increasing) order of their values.
(a) 67 – 19
(b) 67 – 20
(c) 35 + 25
(d) 5 × 11
(e) 120 ÷ 3
Solution:
(a) 67 – 19 = 48
(b) 67 – 20 = 47
(c) 35 + 25 = 60
(d) 5 × 11 = 55
(e) 120 ÷ 3 = 40
Since, 40 < 47 < 48 < 55 < 60
Therefore, 120 ÷ 3 < 67 – 20 < 67 – 19 < 5 × 11 < 35 + 25.
Page 26
Q. Use ‘>’ or ‘<’ or ‘=’ in each of the following expressions to compare them. Can you do it without complicated calculations? Explain your thinking in each case.
(a) 245 + 289 ____ 246 + 285
(b) 273 – 145 ____ 272 – 144
(c) 364 + 587 ____ 363 + 589
(d) 124 + 245 ____ 129 + 245
(e) 213 – 77 ____ 214 – 76
Solution:
(a) 245 + 289 ____ 246 + 285
245 + 1 + 285 + 4 ____ 245 + 1 + 285
245 + 285 + 5 ____ 1 + 245 + 285
Removing the common terms,
5 (>) 1
∴ 245 + 289 (>) 246 + 285.
(b) 273 – 145 ____ 272 – 144
272 + 1 – 144 + 1 ____ 272 – 144
272 – 144 + 2 ____ 272 – 144
Removing the common terms,
2 (>) 0
∴ 273 – 145 (>) 272 – 144.
(c) 364 + 587 ____ 363 + 589
363 + 1 + 587 ____ 363 + 587 + 2
363 + 587 + 1 ____ 2 + 363 + 587
Removing the common terms,
1 (<) 2
∴ 364 + 587 (<) 363 + 589.
(d) 124 + 245 ____ 129 + 245
124 + 245 ____ 124 + 5 + 245
124 + 245 ____ 5 + 124 + 245
Removing the common terms,
0 (<) 5
∴ 124 + 245 (<) 129 + 245.
(e) 213 – 77 ____ 214 – 76
213 – 76 + 1 ____ 213 + 1 – 76
213 – 76 + 1 ____ 213 – 76 + 1
Removing the common terms,
1 (=) 1
∴ 213 – 77 (=) 214 – 76.
Page 28
Q. Check if replacing subtraction by addition in this way does not change the value of the expression, by taking different examples. {Expression: 83 – 14 = 83 +( – 14)}.
Solution:
17 – 9 = 17 + (-9)
8 – 5 = 8 + (-5)
12 – 4 + 6 × 3 = 12 + (-4) + 6 × 3.
Q. Can you explain why subtracting a number is the same as adding its inverse, using the Token Model of integers that we saw in the Class 6 textbook of mathematics?
Solution:
Expression: 8 – 3 = 8 + (-3) or (+8) – (+3) = (+8) + (-3)

Page 29
Q. Complete the table:

Solution:

Q. Does changing the order in which the terms are added give different values?
Solution:
No, changing the order in which the terms are added doesn’t give different values.
Q. Will this also hold when there are terms having negative numbers as well? Take some more expressions and check.
Solution:
No, the sum will not change even on swapping the terms with negative numbers.
Example 1: (-3) + (-9) = -12. Also, (-9) + (-3) = -12.
Example 2: (-5) + 7 = 2. Also, 7 + (-5) = 2.
Page 30
Q. Can you explain why this is happening using the Token Model of integers that we saw in the Class 6 textbook of mathematics?
Solution:
Using token model to explain, (-3) + (-6) = (-6) + (-3) = (-9)
(i) (-3) + (-6) = (-9)

(ii) (-6) + (-3) = (-9)

This proves that the result remains the same even on swapping the positions of the terms with negative numbers.
Q. Will this also hold when there are terms having negative numbers as well? Take some more expressions and check.
Solution:
Yes, even while adding negative integers, grouping them in any order gives the same sum.
Example:
{(-2) + (-5)} + (-7) = (-2) + {(-5) + (-7)}
L.H.S: {(-2) + (-5)} + (-7) = (-7) + (-7) = (-14)
R.H.S: (-2) + {(-5) + (-7)} = (-2) + (-12) = (-14)
Thus, we are getting the same value in both cases.
Q. Can you explain why this is happening using the Token Model of integers that we saw in the Class 6 textbook of mathematics?
Solution:
{(-2) + (-3)} + (-5) = (-2) + {(-3) + (-5)} = (-10)
L.H.S: {(-2) + (-3)} + (-5)

R.H.S: (-2) + {(-3) + (-5)}
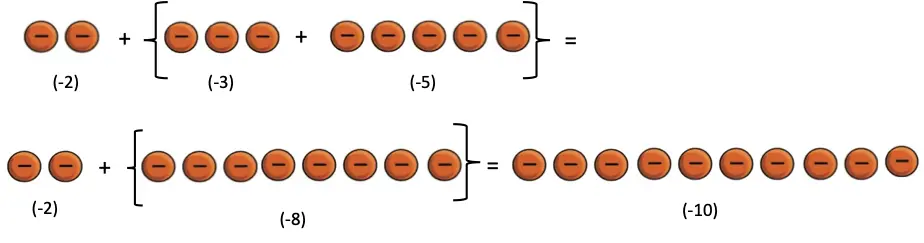
Both L.H.S. and R.H.S. are equal.
Page 31
Q. Does adding the terms of an expression in any order give the same value? Take some more expressions and check. Consider expressions with more than 3 terms also.
Solution:
Yes, adding the terms of an expression in any order gives the same value.
Example:
{(-7) + 12} + (-13) + (-5) = (-7) + 12 + {(-13) + (-5)}
L.H.S: {(-7) + 12} + (-13) + (-5)
= 5 + (-13) + (-5)
= -8 + (-5)
= -13
R.H.S: (-7) + 12 + {(-13) + (-5)}
= (-7) + 12 + (-18)
= 5 + (-18)
= -13
Q. Manasa is adding a long list of numbers. It took her five minutes to add them all and she got the answer 11749. Then she realised that she had forgotten to include the fourth number 9055. Does she have to start all over again? (Numbers list: 1342, 774, 8611, 9055, 1022)
Solution:
No, she doesn’t have to start over again. She can simply add the forgotten number (9055) to the previous sum (11749) to get the final correct answer.
Calculation: 11,749 + 9,055 = 20,804.
Q. Manasa is going outside to play. Her mother says, “Wear your hat and shoes!” Which one should she wear first?
Solution:
It doesn’t matter what she wears first; both pieces contribute equally to her final look. This situation is commutative.
Page 32
Q. If the total number of friends goes up to 7 and the tip remains the same, how much will they have to pay? Write an expression for this situation and identify its terms. (Amu, Charan, Madhu, and John went to a hotel and ordered four dosas. Each dosa cost ₹23, and they wish to thank the waiter by tipping ₹5.)
Solution:
Cost of each dosa = ₹23
Cost of 7 dosas = 7 × ₹23
Tip given = ₹5
Expression for total amount with tip = (7 × ₹23) + ₹5
= ₹161 + ₹5
= ₹166.
Terms in expression: (7 × ₹23), ₹5.
Q. Children playing “Fire in the mountain”. 33 students were playing and ruby didn’t play. Teacher called out ‘5’. Students formed groups of 5. Ruby wrote 6 x 5 + 3. Think and discuss why she wrote this.
Solution:
Ruby wrote 6 × 5 + 3 because the expression (6 × 5) represents the total number of students who grouped themselves in teams of 5, and 3 represents the students who were left over, as they couldn’t form a complete group of 5.
Page 33
Q. For each of the cases below, write the expression and identify its terms:
(i) If the teacher had called out ‘4’, Ruby would write ________
(ii) If the teacher had called out ‘7’, Ruby would write ________
(iii) Write expressions like the above for your class size.
Solution:
(i) Since 33 ÷ 4 gives a quotient of 8 and a remainder of 1. Therefore, Ruby could write ( 4 × 8 + 1 ).
(ii) Since 33 ÷ 7 gives a quotient of 4 and a remainder of 5. Therefore, Ruby could write ( 7 × 4 + 5 ).
(iii) Number of students in my class = 43
If the teacher had called out 4, the expression would be ( 4 × 10 + 3 ).
If the teacher had called out 7, the expression would be ( 7 × 6 + 1 ).
Q. Identify the terms in the two expressions above.
(Expressions: 432 = 4 × 100 + 1 × 20 + 1 × 10 + 2 × 1 and 432 = 8 × 50 + 1 × 10 + 4 × 5 + 2 × 1)
Solution:
(i) 432 = 4 × 100 + 1 × 20 + 1 × 10 + 2 × 1
Terms: (4 × 100), (1 × 20), (1 × 10), and (2 × 1).
(ii) 432 = 8 × 50 + 1 × 10 + 4 × 5 + 2 × 1
Terms: (8 × 50), (1 × 10), (4 × 5), and (2 × 1).
Q. Can you think of some more ways of giving ₹432 to someone?
Solution:
Some ways are:
(i) 432 = 8 × 50 + 3 × 10 + 2 × 1.
(ii) 432 = 20 × 20 + 2 × 10 + 2 × 5 + 2 × 1.
Page 34
Figure it out
1. Find the values of the following expressions by writing the terms in each case.
(a) 28 – 7 + 8
(b) 39 – 2 × 6 + 11
(c) 40 – 10 + 10 + 10
(d) 48 – 10 × 2 + 16 ÷ 2
(e) 6 × 3 – 4 × 8 × 5
Solution:
(a) 28 – 7 + 8 = 28 + (-7) + 8
= 21 + 8 = 29.
Terms: 28, (-7), 8.
(b) 39 – 2 × 6 + 11 = 39 + {(-2) × 6} + 11
= 39 +(-12) + 11
= 27 + 11 =38.
Terms: 39, (-2 × 6), 11.
(c) 40 – 10 + 10 + 10 = 40 + (-10) + 10 + 10
= 30 + 10 + 10
= 30 + 20 = 50.
Terms: 40, (-10), 10, 10.
(d) 48 – 10 × 2 + 16 ÷ 2 = 48 + (-10 × 2) + (16 × 2)
= 48 + (-20) + 8
= 28 + 8 = 36.
Terms: 48, (-10 × 2), (16 × 2).
(e) 6 × 3 – 4 × 8 × 5 = (6 × 3) + (-4 × 8 × 5)
= 18 + (-160)
= -142.
Terms: (6 × 3), (-4 × 8 × 5).
2. Write a story/situation for each of the following expressions and find their values.
(a) 89 + 21 – 10 (b) 5 × 12 – 6
(c) 4 × 9 + 2 × 6
Solution:
(a) Story: Ramesh had 89 marbles. He got 21 more marbles from his friend and later gave 10 marbles to another friend.
Expression: 89 + 21 – 10 = 110 – 10 = 100.
(b) Story: There were 5 classrooms, each having 12 desks. One day, 6 desks were found broken and were removed.
Expression: 5 × 12 – 6 = 60 – 6 = 54.
(c) Story: A bakery baked 4 trays of cupcakes, with 9 cupcakes on each tray, and 2 trays of cookies, with 6 cookies on each tray.
Expression: 4 × 9 + 2 × 6 = 36 + 12 = 48.
3. For each of the following situations, write the expression describing the situation, identify its terms and find the value of the expression.
(a) Queen Alia gave 100 gold coins to Princess Elsa and 100 gold coins to Princess Anna last year. Princess Elsa used the coins to start a business and doubled her coins. Princess Anna bought jewellery and has only half of the coins left. Write an expression describing how many gold coins Princess Elsa and Princess Anna together have.
(b) A metro train ticket between two stations is ₹40 for an adult and ₹20 for a child. What is the total cost of tickets:
(i) for four adults and three children?
(ii) for two groups having three adults each?
(c) Find the total height of the window by writing an expression describing the relationship among the measurements shown in the picture.

Solution:
(a) Princess Elsa and Princess Anna both had 100 gold coins each.
Elsa doubled her coins, while Anna was left with half of hers.
Expression: (2 × 100) + (100 ÷ 2)
Terms: (2 × 100), (100 × 2).
Value: (2 × 100) + (100 ÷ 2) = 200 + 50 = 250.
Therefore, together they have 250 gold coins.
(b) Ticket for adults = ₹40
Ticket for a child = ₹20
(i) For four adults and three children:
Expression: (4 × 40) + (3 × 20)
Terms: (4 × 40), (3 × 20)
Value: (4 × 40) + (3 × 20) = 160 + 60 = 220.
Therefore, the total cost is ₹220.
(ii) For two groups having three adults each:
Expression: (3 × 40) + (3 × 40)
Terms: (3 × 40), (3 × 40)
Value: (3 × 40) + (3 × 40) = 120 + 120 = 240.
Therefore, the total cost is ₹240.
(c) Border = 3 cm
Grill = 2 cm
Gap = 5 cm
Expression: (6 × 2) + (2 × 3) + (7 × 5)
Terms: (6 × 2), (2 × 3), (7 × 5).
Value: (6 × 2) + (2 × 3) + (7 × 5) = 12 + 6 + 35 = 53.
Page 37
Q.

Solution:

Figure it out
1. Fill in the blanks with numbers, and boxes with operation signs such that the expressions on both sides are equal.
(a) 24 + (6 – 4) = 24 + 6 ☐ _____
(b) 38 + ( _____ ☐ _____ ) = 38 + 9 – 4
(c) 24 – (6 + 4) = 24 ☐ 6 – 4
(d) 24 – 6 – 4 = 24 – 6 ☐ _____
(e) 27 – (8 + 3) = 27 ☐ 8 ☐ 3
(f) 27 – ( _____ ☐ _____ ) = 27 – 8 + 3
Solution:

2. Remove the brackets and write the expression having the same value.
(a) 14 + (12 + 10)
(b) 14 – (12 + 10)
(c) 14 + (12 – 10)
(d) 14 – (12 – 10)
(e) –14 + 12 – 10
(f) 14 – (–12 – 10)
Solution:
(a) 14 + (12 + 10) = 14 + 12 + 10
(b) 14 – (12 + 10) = 14 – 12 – 10
(c) 14 + (12 – 10) = 14 + 12 – 10
(d) 14 – (12 – 10) = 14 – 12 + 10
(e) -14 + 12 – 10 = -14 + 12 – 10
(f) 14 – (-12 – 10) = 14 + 12 + 10
3. Find the values of the following expressions. For each pair, first try to guess whether they have the same value. When are the two expressions equal?
(a) (6 + 10) – 2 and 6 + (10 – 2)
(b) 16 – (8 – 3) and (16 – 8) – 3
(c) 27 – (18 + 4) and 27 + (–18 – 4)
Solution:
(a) (6 + 10) – 2 and 6 + (10 – 2)
6 + 10 – 2 _____ 6 + 10 – 2
16 – 2 _____ 16 – 2
14 (=) 14
Here, the two expressions are equal.
(b) 16 – (8 – 3) and (16 – 8) – 3
16 – 8 + 3 _____ 16 – 8 – 3
8 + 3 _____ 8 – 3
11 (≠) 4
Here, the two expressions are not equal.
(c) 27 – (18 + 4) and 27 + (–18 – 4)
27 – 18 – 4 _____ 27 – 18 – 4
9 – 4 _____ 9 – 4
5 (=) 5
Here, the two expressions are equal.
Page 38
4. In each of the sets of expressions below, identify those that have the same value. Do not evaluate them, but rather use your understanding of terms.
(a) 319 + 537, 319 – 537, –537 + 319, 537 – 319
(b) 87 + 46 – 109, 87 + 46 – 109, 87 + 46 – 109, 87 – 46 + 109, 87 – (46 + 109), (87 – 46) + 109
Solution:
(a) 319 + 537, 319 – 537, –537 + 319, 537 – 319
Same value → 319 – 537 and –537 + 319 → Follow the commutative property of addition.
(b) 87 + 46 – 109, 87 + 46 – 109, 87 + 46 – 109, 87 – 46 + 109, 87 – (46 + 109), (87 – 46) + 109
Same value → 87 – 46 + 109 and (87 – 46) + 109 → Follow the associative property of addition.
Exactly same → 87 + 46 – 109, 87 + 46 – 109, 87 + 46 – 109.
5. Add brackets at appropriate places in the expressions such that they lead to the values indicated.
(a) 34 – 9 + 12 = 13
(b) 56 – 14 – 8 = 34
(c) –22 – 12 + 10 + 22 = –22
Solution:
(a) 34 – 9 + 12 = 13
34 – (9 + 12) = 13.
(b) 56 – 14 – 8 = 34
(56 – 14) – 8 = 34
(c) –22 – 12 + 10 + 22 = –22
–22 – (12 + 10) + 22 = –22
6. Using only reasoning of how terms change their values, fill the blanks to make the expressions on either side of the equality (=) equal.
(a) 423 + ____ = 419 + ____
(b) 207 – 68 = 210 – ____
Solution:
(a) 423 + ____ = 419 + ____
Since 419 is 4 less than 423, we must add 4 more to 419’s side to balance.
∴ 433 + 2 = 419 + 6. (6 is more than 2).
(b) 207 – 68 = 210 – ____
Since 210 is 3 more than 207, subtract 3 more from right side to balance.
∴ 207 – 68 = 210 – 71 (71 is 3 more than 68)
7. Using the numbers 2, 3 and 5, and the operators ‘+’ and ‘–’, and brackets, as necessary, generate expressions to give as many different values as possible. For example, 2 – 3 + 5 = 4 and 3 – (5 – 2) = 0.
Solution:
(i) 2 + (3 + 5) = 10
(ii) 2 – (3 + 5) = 2 – 8 = -6
(iii) (-2) – (3 + 5) = -2 – 8 = -10
(iv) (-2) + (3 – 5) = -2 + (-2) = -4
(v) 5 + (3 – 2) = 5 + 1 = 6
Different values obtained are 10, -10, 6, -6, -4.
8. Whenever Jasoda has to subtract 9 from a number, she subtracts 10 and adds 1 to it. For example, 36 – 9 = 26 + 1.
(a) Do you think she always gets the correct answer? Why?
(b) Can you think of other similar strategies? Give some examples.
Solution:
(a) Yes, Jasoda always gets the correct answer.
Subtracting 9 is the same as subtracting 10 and adding 1 back, because:
–9 = –10 + 1
So, 36 – 9 = 36 – 10 + 1 = 26 + 1 = 27, which is correct.
(b) Examples of some similar strategies:-
To subtract 19, subtract 20 and add 1:
54 – 19 = 54 – 20 + 1 = 34 + 1 = 35
To subtract 8, subtract 10 and add 2:
43 – 8 = 43 – 10 + 2 = 33 + 2 = 35
To subtract 18, subtract 20 and add 2:
65 – 18 = 65 – 20 + 2 = 45 + 2 = 47
9. Consider the two expressions: a) 73 – 14 + 1, b) 73 – 14 – 1. For each of these expressions, identify the expressions from the following collection that are equal to it.
(a) 73 – (14 + 1) (b) 73 – (14 – 1) (c) 73 + (–14 + 1) d) 73 + (–14 – 1)
Solution:
a) 73 – 14 + 1 = 59 + 1 = 60.
b) 73 – 14 – 1 = 59 – 1 = 58.
(a) 73 – (14 + 1) = 73 – 15 = 58.
(b) 73 – (14 – 1) = 73 – 13 = 60.
(c) 73 + (–14 + 1) = 73 + (-13) = 60.
(d) 73 + (-14 – 1) = 73 + (-15) = 58.
Therefore,
73 – 14 + 1 is equal to 73 – (14 – 1) and 73 + (–14 + 1).
73 – 14 – 1 is equal to 73 – (14 + 1) and 73 + (-14 – 1).
Page 39
Q. If another friend, Sangmu, joins them and orders the same items, what will be the expression for the total amount to be paid? [Expression for Lhamo and Norbu who each ordered a vegetable cutlet costing ₹43 and a rasgulla costing ₹24 is 2 × (43 + 24)]
Solution:
If another friend, Sangmu joins them and orders the same items.
Then, the total amount to be paid is 3 × (43 + 24).
Page 40
Q. 5 × 4 + 3 ≠ 5 × (4 + 3). Can you explain why?
Solution:
L.H.S: 5 × 4 + 3 = 20 + 3 = 23
R.H.S: 5 × (4 + 3) = 5 × 4 + 5 × 3 = 20 + 15 = 35.
Since, 23 ≠ 35
∴ 5 × 4 + 3 ≠ 5 × (4 + 3).
Q. Is 5 × (4 + 3) = 5 × (3 + 4) = (3 + 4) × 5?
Solution:
5 × (4 + 3) = 5 × 4 + 5 × 3 = 20 + 15 = 35.
5 × (3 + 4) = 5 × 3 + 5 × 4 = 15 + 20 = 35.
(3 + 4) × 5 = 7 × 5 = 35.
Since, all these expressions have 35 as result.
∴ 5 × (4 + 3) = 5 × (3 + 4) = (3 + 4) × 5.
Page 41
Q. 97 × 25 = 100 × 25 – 3 × 25. Find this value.
Solution:
97 × 25 = 100 × 25 – 3 × 25 = 2500 – 75 = 2425.
Q. Use this method to find the following products:
(a) 95 × 8
(b) 104 × 15
(c) 49 × 50
Is this quicker than the multiplication procedure you use generally?
Solution:
(a) 95 × 8 = 95 × (10 – 2)
= 95 × 10 – 95 × 2
= 950 – 190 = 760.
(b) 104 × 15 = (100 + 4) × 15
= 100 × 15 + 4 × 15
= 1500 + 60 = 1560.
(c) 49 × 50 = (50 – 1) × 50
= 50 × 50 – 1 × 50
= 2500 – 50 = 2450.
Q. Which other products might be quicker to find like the ones above?
Solution:
(i) 36 × 102 = 36 × (100 + 2) = 36 × 100 + 36 × 2 = 3600 + 72 = 3672.
(ii) 75 × 98 = 75 × (100 – 2) = 75 × 100 – 75 × 2 = 7500 – 150 = 7350.
(iii) 42 × 52 = 42 × (50 + 2) = 42 × 50 + 42 × 2 = 2100 + 84 = 2184.
(iv) 995 × 67 = (1000 – 5) × 67 = 67 × 1000 – 67 × 5 = 6700 – 335 = 6365.
Figure it out
1. Fill in the blanks with numbers, and boxes by signs, so that the expressions on both sides are equal.
(a) 3 × (6 + 7) = 3 × 6 + 3 × 7
(b) (8 + 3) × 4 = 8 × 4 + 3 × 4
(c) 3 × (5 + 8) = 3 × 5 ☐ 3 × ____
(d) (9 + 2) × 4 = 9 × 4 ☐2 × ____
(e) 3 × (____ + 4) = 3 ____ + ____
(f) (____ + 6) × 4 = 13 × 4 + ____
(g) 3 × ( ____ + ____ ) = 3 × 5 + 3 × 2
(h) ( ____ + ____ ) × ____ = 2 × 4 + 3 × 4
(i) 5 × (9 – 2) = 5 × 9 – 5 × ____
(j) (5 – 2) × 7 = 5 × 7 – 2 × ____
(k) 5 × (8 – 3) = 5 × 8 ☐ 5 × ____
(l) (8 – 3) × 7 = 8 × 7 ☐ 3 × 7
(m) 5 × (12 – ____ ) = ____ ☐ 5 × ____
(n) (15 – ____ ) × 7 = ____ ☐ 6 × 7
(o) 5 × ( ____ + ____ ) = 5 × 9 – 5 × 4
(p) ( ____ + ____ ) × ___ = 17 × 7 – 9 × 7
Solution:

Page 42
2. In the boxes below, fill ‘<’, ‘>’ or ‘=’ after analysing the expressions on the LHS and RHS. Use reasoning and understanding of terms and brackets to figure this out and not by evaluating the expressions.
(a) (8 – 3) x 29 ____ (3 – 8) x 29
(b) 15 + 9 x 18 ____ (15 + 9) x 18
(c) 23 x (17 – 9) ____ 23 x 17 + 23 x 9
(d) (34 – 28) x 42 ____ 34 x 42 – 28 x 42
Solution:
(a) (8 – 3) x 29 _ (3 – 8) x 29
Positive x 29 (>) Negative x 29
∴ (8 – 3) x 29 (>) (3 – 8) x 29.
(b) 15 + 9 x 18 _ (15 + 9) x 18
Smaller value (multiplication first) (<) Bigger Value (addition first)
∴ 15 + 9 x 18 (<) (15 + 9) x 18.
(c) 23 x (17 – 9) _ 23 x 17 + 23 x 9
Smaller value (involves subtraction of terms) (<) Bigger value (involves addition of terms)
23 x (17 – 9) (<) 23 x 17 + 23 x 9.
(d) (34 – 28) x 42 _ 34 x 42 – 28 x 42
This is a property called distributive property, so both are equal.
(34 – 28) x 42 (=) 34 x 42 – 28 x 42.
3. Here is one way to make 14: 2 x (1 + 6) = 14. Are there other ways of getting 14? Fill them out below:
(a) ____ × (____ + ____) = 14
(b) ____ × (____ + ____) = 14
(c) ____ × (____ + ____) = 14
(d) ____ × (____ + ____) = 14
Solution:
(a) 2 × (3 + 4) = 14
(b) 7 × (2 + 0) = 14
(c) 2 × (5 + 2) = 14
(d) 2 × (0 + 7) = 14
4. Find out the sum of the numbers given in each picture below in at least two different ways. Describe how you solved it through expressions.
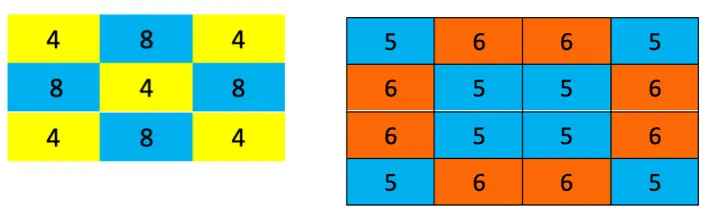
Solution:
(i) Sum = 4 × 5 + 8 × 4 = 20 + 32 = 52.
Also, Sum = 4 × (5 + 8) = 4 × 13 = 52.
(ii) Sum = 5 × 8 + 6 × 8 = 40 + 48 = 88.
Also, Sum = 8 × (5 + 6) = 8 × (11) = 88.
Figure it out
1. Read the situations given below. Write appropriate expressions for each of them and find their values.
(a) The district market in Begur operates on all seven days of a week. Rahim supplies 9 kg of mangoes each day from his orchard and Shyam supplies 11 kg of mangoes each day from his orchard to this market. Find the amount of mangoes supplied by them in a week to the local district market.
(b) Binu earns ₹20,000 per month. She spends ₹5,000 on rent, ₹5,000 on food, and ₹2,000 on other expenses every month. What is the amount Binu will save by the end of a year?
(c) During the daytime a snail climbs 3 cm up a post, and during the night while asleep, accidentally slips down by 2 cm. The post is 10 cm high, and a delicious treat is on its top. In how many days will the snail get the treat?
Solution:
(a) Mangoes supplied by Rahim per day = 9 kg
Mangoes supplied by Shyam per day = 11 kg
Total amount of mangoes supplied by both in a week = 7 × (9 + 11) kg
= 7 × 9 + 7 × 11
= 63 + 77 = 140 kg.
(b) Total salary = ₹20,000
Rent expenses = ₹5,000
Food expenses = ₹5,000
Other expenses = ₹2,000
Total amount saved at the end of year = 12 × {20,000 – (5000 + 5000 + 2000)}
= 12 × {20,000 – 12,000}
= 12 × 8,000 = ₹96,000.
(c) Height of post = 10 cm
Climb during the day = 3 cm
Slip during the night = 2 cm
Let, total days taken by snail = y
∴ 10 = y × (3 – 2)
10 = y × 1
y = 10.
Therefore, the snail will take 10 days to reach the top.
Page 43
2. Melvin reads a two-page story every day except on Tuesdays and Saturdays. How many stories would he complete reading in 8 weeks? Which of the expressions below describes this scenario?
(a) 5 × 2 × 8
(b) (7 – 2) × 8
(c) 8 × 7
(d) 7 × 2 × 8
(e) 7 × 5 – 2
(f) (7 + 2) × 8
(g) 7 × 8 – 2 × 8
(h) (7–5) × 8
Solution:
Stories completed in 8 weeks are represented by the expressions given below:
(b) (7 – 2) × 8
(g) 7 × 8 – 2 × 8
3. Find different ways of evaluating the following expressions:
(a) 1 – 2 + 3 – 4 + 5 – 6 + 7 – 8 + 9 – 10
(b) 1 – 1 + 1 – 1 + 1 – 1 + 1 – 1 + 1 – 1
Solution:


4. Compare the following pairs of expressions using ‘<’, ‘>’ or ‘=’ or by reasoning.
(a) 49 – 7 + 8 ____ 49 – 7 + 8
(b) 83 x 42 – 18 ____ 83 x 40 – 18
(c) 145 – 17 x 8 ____ 145 – 17 x 6
(d) 23 x 48 – 35 ____ 23 x (48 – 35)
(e) (16 – 11) x 12 ____ -11 x 12 + 16 x 12
(f) (76 – 53) x 88 ____ 88 x (53 – 76)
(g) 25 x (42 + 16) ____ 25 x (43 + 15)
(h) 36 x (28 – 16) ____ 35 x (27 – 15)
Solution:
(a) 49 – 7 + 8 ____ 49 – 7 + 8
Both sides are exactly the same.
∴ 49 – 7 + 8 (=) 49 – 7 + 8
(b) 83 x 42 – 18 ____ 83 x 40 – 18
3486 – 18 ____ 3320 – 18
3468 (>) 3302.
∴ 83 x 42 – 18 (>) 83 x 40 – 18
(c) 145 – 17 x 8 ____ 145 – 17 x 6
145 – 136 ____ 145 – 102
9 (<) 43
∴ 145 – 17 x 8 (<) 145 – 17 x 6
(d) 23 x 48 – 35 ____ 23 x (48 – 35)
1104 – 35 ____ 23 x 13
1069 (>) 299
∴ 23 x 48 – 35 (>) 23 x (48 – 35)
(e) (16 – 11) x 12 ____ -11 x 12 + 16 x 12
5 x 12 ____-132 + 192
60 (=) 60
∴ (16 – 11) x 12 (=) -11 x 12 + 16 x 12
(f) (76 – 53) x 88 ____ 88 x (53 – 76)
23 x 88 ____ 88 x -23
2024 (>) -2024
∴ (76 – 53) x 88 (>) 88 x (53 – 76)
(g) 25 x (42 + 16) ____ 25 x (43 + 15)
25 x 58 ____ 25 x 58
1450 (=) 1450
∴ 25 x (42 + 16) (=) 25 x (43 + 15)
(h) 36 x (28 – 16) ____ 35 x (27 – 15)
36 x 12 ____ 35 x 12
432 (>) 420
∴ 36 x (28 – 16) (>) 35 x (27 – 15)
5. Identify which of the following expressions are equal to the given expression without computation. You may rewrite the expressions using terms or removing brackets. There can be more than one expression which is equal to the given expression.
(a) 83 – 37 – 12
(i) 84 – 38 – 12
(ii) 84 – (37 + 12)
(iii) 83 – 38 – 13
(iv) -37 + 83 – 12
(b) 93 + 37 × 44 + 76
(i) 37 + 93 x 44 + 76
(ii) 93 + 37 x 76 + 44
(iii) (93 + 37) x (44 + 76)
(iv) 37 x 44 + 93 + 76
Solution:
(a) 83 – 37 – 12
(i) 84 – 38 – 12 contains different terms than the given expression. Therefore, it is not equal to the original expression.
(ii) 84 – (37 + 12) = 84 – 37 – 12 which is same as the given expression.
(iii) 83 – 38 – 13 contains different terms than the given expression. Therefore, it is not equal to the original expression.
(iv) -37 + 83 – 12 = 83 – 37 – 12 which is same as the given expression.
∴ (a) is equal to (ii) and (iv).
(b) 93 + 37 × 44 + 76 = 93 + (37 × 44) + 76
(i) 37 + 93 x 44 + 76 = 37 + (93 x 44) + 76. The multiplication uses different numbers than the given expression. Therefore, it is not equal to the original expression.
(ii) 93 + 37 x 76 + 44 = 93 + (37 x 76) + 44. The multiplication uses different numbers than the given expression. Therefore, it is not equal to the original expression.
(iii) (93 + 37) x (44 + 76). The grouping and operation order are different than the given expression. Therefore, it is not equal to the original expression.
(iv) 37 x 44 + 93 + 76 = (37 × 44) + 93 + 76 which can be rearranged as 93 + (37 × 44) + 76.
Therefore, it is equal to the original expression.
∴ (b) is equal to (iv).
5. Choose a number and create ten different expressions having that value.
Solution:
Number chosen: 7
(i) 3 + (9 – 5)
(ii) (10 – 5) + 2
(iii) 2 x 3 + 1
(iv) (11 – 8) + (15 – 11)
(v) (8 × 2) – 9
(vi) 5 + (6 – 4)
(vii) 2 + (9 – 4)
(viii) (12 × 2) – 17
(ix) 3 + (8 – 4)
(x) (7 × 2) – 7
